A fully-discrete finite element approximation for the eddy currents problem
Main Article Content
Keywords
Transient eddy current model, potential formulation, fully-discrete approximation, finite elements, error estimates
Abstract
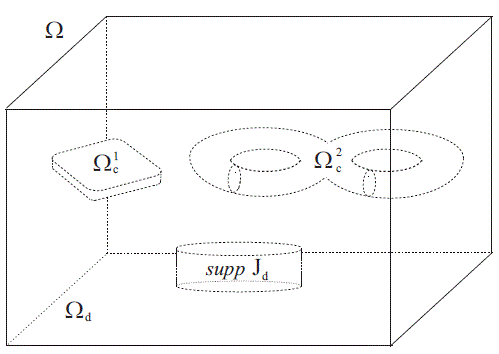
The eddy current model is obtained from Maxwell’s equations by neglecting the displacement currents in the Amp`ere-Maxwell’s law and it is commonly used in many problems in sciences, engineering and industry (e.g, in induction heating, electromagnetic braking, and power transformers). The so-called “A, V −A potential formulation” (B´ır´o & Preis [1]) is nowadays one of the most accepted formulations to solve the eddy current equations numerically, and B´ır´o & Valli [2] have recently provided its well-posedness and convergence analysis for the time-harmonic eddy current problem. The aim of this paper is to extend the analysis performed by B´ır´o & Valli to the general transient eddy current model. We provide a backward-Euler fully-discrete approximation based on nodal finite elements and we show that the resulting discrete variational problem is well posed. Furthermore, error estimates that prove optimal convergence are settled.
MSC: 78M10, 65N30
Downloads
References
[2] A. Bossavit, Computational electromagnetism, ser. Electromagnetism. San Diego, CA: Academic Press Inc., 1998. 112
[3] H. Ammari, A. Buffa, and J.-C. Nédélec, “A justification of eddy currents model for the Maxwell equations,” SIAM Journal on Applied Mathematics, vol. 60, no. 5, pp. 1805–1823, 2000. 113
[4] A. Alonso Rodríguez and A. Valli, Eddy current approximation of Maxwell equations, ser. MS&A. Modeling, Simulation and Applications. Springer-Verlag Italia, Milan, 2010, vol. 4. [Online]. Available:
http://dx.doi.org/10.1007/978-88-470-1506-7 113, 124
[5] S. Meddahi and V. Selgas, “An H-based FEM-BEM formulation for a time dependent eddy current problem,” Applied Numerical Mathematics, vol. 58, no. 8, pp. 1061–1083, 2008. 113, 124
[6] C. Ma, “The finite element analysis of a decoupled T-Φ scheme for solving eddycurrent problems,” Applied Mathematics and Computation, vol. 205, no. 1, pp. 352–361, 2008. 113
[7] R. Acevedo, S. Meddahi, and R. Rodríguez, “An E-based mixed formulation for a time-dependent eddy current problem,” 2009. 113, 124
[8] T. Kang and K. Kim, “Fully discrete potential-based finite element methods for a transient eddy current problem,” Computing, vol. 85, no. 4, pp. 339–362, 2009. 113
[9] R. A. Prato Torres, E. P. Stephan, and F. Leydecker, “A FE/BE coupling for the 3D time-dependent eddy current problem. Part I: a priori error estimates,” Computing, vol. 88, no. 3-4, pp. 131–154, 2010. [Online]. Available: http://dx.doi.org/10.1007/s00607-010-0089-9 113
[10] R. Acevedo and S. Meddahi, “An E-based mixed FEM and BEM coupling for a time-dependent eddy current problem,” IMA Journal of Numerical Analysis, vol. 31, no. 2, pp. 667–697, 2011. 113, 124
[11] A. Bermúdez, R. López, R. Rodríguez, and P. Salgado, “Numerical solution of transient eddy current problems with input current intensities as boundary data,” IMA Journal of Numerical Analysis, vol. 32, pp. 1001–1029, 2012. 113
[12] J. Camaño and R. Rodríguez, “Analysis of a FEM-BEM model posed on the conducting domain for the time-dependent eddy current problem,” J. Comput. Appl. Math., vol. 236, no. 13, pp. 3084–3100, 2012. [Online]. Available:http://dx.doi.org/10.1016/j.cam.2012.01.030 113
[13] O. Bíró and K. Preis, “On the use of the magnetic vector potential in the finiteelement analysis of three-dimensional eddy currents,” Magnetics, IEEE Transactions on, vol. 25, no. 4, pp. 3145–3159, 1989. 113, 120, 121, 124
[14] O. Bíró and A. Valli, “The Coulomb gauged vector potential formulation for the eddy-current problem in general geometry: wellposedness and numerical approximation,” Comput. Methods Appl. Mech.
Engrg., vol. 196, no. 13-16, pp. 1890–1904, 2007. [Online]. Available: http://dx.doi.org/10.1016/j.cma.2006.10.008 113, 114, 121, 124, 127
[15] A. Bermúdez, R. Rodríguez, and P. Salgado, “FEM for 3D eddy current problems in bounded domains subject to realistic boundary conditions. An application to metallurgical electrodes,” Computer Methods in Applied Mechanics and Engineering, vol. 12, no. 1, pp. 67–114, 2005. 113, 124
[16] C. Yongbin, Y. Junyou, Y. Hainian, and T. Renyuan, “Study on eddy current losses and shielding measures in large power transformers,” Magnetics, IEEE Transactions on, vol. 30, no. 5, pp. 3068–3071, 1994. 113
[17] R. Acevedo and R. Rodríguez, “Analysis of the A, V-A- potential formulation for the eddy current problem in a bounded domain,” Electron. Trans. Numer. Anal., vol. 26, pp. 270–284, 2007. 114, 140
[18] P. Monk, Finite element methods for Maxwell’s equations, ser. Numerical Mathematics and Scientific Computation. New York: Oxford University Press, 2003. [Online]. Available: http://dx.doi.org/10.1093/acprof:oso/9780198508885.001.0001 115, 116, 118
[19] A. Buffa and P. Ciarlet Jr., “On traces for functional spaces related to Maxwell’s equations. I. An integration by parts formula in Lipschitz polyhedra,” Mathematical Methods in the Applied Sciences, vol. 24, no. 1, pp. 9–30, 2001. 116, 117
[20] ——, “On traces for functional spaces related to Maxwell’s equations. II. Hodge decompositions on the boundary of Lipschitz polyhedra and applications,” Mathematical Methods in the Applied Sciences, vol. 24, no. 1, pp. 31–48, 2001. 116
[21] A. Buffa, M. Costabel, and D. Sheen, “On traces for H(curl,Ω) in Lipschitz domains,” J. Math. Anal. Appl., vol. 276, no. 2, pp. 845–867, 2002. [Online]. Available: http://dx.doi.org/10.1016/S0022-47X(02)00455-9 116, 117
[22] E. Zeidler, Nonlinear functional analysis and its applications. II/A. New York: Springer-Verlag, 1990. [Online]. Available: http://dx.doi.org/10.1007/978-1-4612-0985-0 119
[23] V. Girault and P.-A. Raviart, Finite element methods for Navier-Stokes equations, ser. Springer Series in Computational Mathematics. Berlin: Springer-Verlag, 1986, vol. 5. [Online]. Available:
http://dx.doi.org/10.1007/978-3-642-61623-5 121, 124, 129
[24] C. Amrouche, C. Bernardi, M. Dauge, and V. Girault, “Vector potentials in three-dimensional non-smooth domains,” Mathematical Methods in the Applied Sciences, vol. 21, no. 9, pp. 823–864, 1998. 124, 129
[25] D. Boffi, F. Brezzi, L. F. Demkowicz, R. G. Durán, R. S. Falk, and M. Fortin, Mixed finite elements, compatibility conditions, and applications, ser. Lecture Notes in Mathematics. Berlin: Springer-Verlag, 2008, vol. 1939. [Online]. Available: http://dx.doi.org/10.1007/978-3-540-78319-0 124
[26] A. Bermúdez, R. Rodríguez, and P. Salgado, “A finite element method with Lagrange multipliers for low-frequency harmonic Maxwell equations,” SIAM J. Numer. Anal., vol. 40, no. 5, pp. 1823–1849, 2002. [Online]. Available: http://dx.doi.org/10.1137/S0036142901390780 124
[27] M. Zlámal, “Finite Element Solution of Quasistationary Nonlinear Magnetic Field,” Rairo-Analyse numérique, vol. 16, no. 2, pp. 161–191, 1982. 126, 127
[28] ——, “Addendum to the paper ’Finite element solution of quasistationary nonlinear magnetic field’,” RAIRO Anal. Numér., vol. 17, no. 4, pp. 407–415, 1983. 126, 127
[29] R. E. Showalter, Monotone operators in Banach space and nonlinear partial differential equations, ser. Mathematical Surveys and Monographs. Providence, RI: American Mathematical Society, 1997, vol. 49. 127
[30] J. Jin, The Finite Element Method in Electromagnetics, 2nd ed. New York: Wiley, 2002. 127
[31] A. Quarteroni and A. Valli, Numerical approximation of partial differential equations, ser. Springer Series in Computational Mathematics. Berlin: Springer-Verlag, 1994, vol. 23. 133
[32] P. G. Ciarlet, The finite element method for elliptic problems, ser. Classics in Applied Mathematics. Philadelphia, PA: Society for Industrial and Applied Mathematics (SIAM), 2002, vol. 40. [Online]. Available:
http://dx.doi.org/10.1137/1.9780898719208 140
[33] M. Costabel and M. Dauge, “Singularities of electromagnetic fields in polyhedral domains,” Arch. Ration. Mech. Anal., vol. 151, no. 3, pp. 221–276, 2000. [Online]. Available: http://dx.doi.org/10.1007/s002050050197 140
[34] M. Costabel, M. Dauge, and S. Nicaise, “Singularities of eddy current problems,” M2AN Math. Model. Numer. Anal., vol. 37, no. 5, pp. 807–831, 2003. [Online]. Available: http://dx.doi.org/10.1051/m2an:2003056 140
[35] M. Costabel and M. Dauge, “Weighted regularization of Maxwell equations in polyhedral domains. A rehabilitation of nodal finite elements,” Numer. Math., vol. 93, no. 2, pp. 239–277, 2002. [Online]. Available:
http://dx.doi.org/10.1007/s002110100388 140
[36] O. Bíró, “Edge element formulations of eddy current problems,” Comput. Methods Appl. Mech. Engrg., vol. 169, no. 3-4, pp. 391–405, 1999. [Online]. Available: http://dx.doi.org/10.1016/S0045-7825(98)00165-0 140