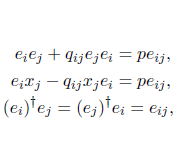e— Calculus
Main Article Content
Keywords
e—derivative, e— algebra, e-integral, q — e algebra
Abstract
In this work formulate the e— calculus based on the nature of the electric charges, using Newton third law and the Coulomb law, the e— algebra and the q — e deformed algebra associating the variables ei; ej as elementary charges, and x as the conductive variable. The e— derivative is defined from a simple experiment off-on light bulb respectively. On the other hand, the e— series, the e— integral, the q — e derivatives, series and integrals and their respective convergence criteria are formulated. On the e— integrals a path or closed contour Γ (x) is established to define the e— contour integrals and finally the q — e deformed calculus and the q — e Heisenberg algebra are formulated.
Downloads
References
[2] C. A. Coulomb, Premier-[troisième] mémoire sur l’electricité et le magnétisme. Académie Royale des sciences, 1785.
[3] W. R. Hamilton, “On quaternions; or on a new system of imaginaries in algebra,” Philosophical magazine, vol. 25, no. 3, pp. 489–495, 1844. https://www.emis.de/classics/Hamilton/OnQuat.pdf
[4] H. Grassmann, Die lineale Ausdehnungslehre ein neuer Zweig der Mathematik: dargestellt und durch Anwendungen auf die übrigen Zweige der Mathematik, wie auch auf die Statik, Mechanik, die Lehre vom Magnetismus und die Krystallonomie erläutert. O. Wigand, 1844, vol. 1.
[5] F. H. Jackson, “Xi.—on q-functions and a certain difference operator,” Earth and Environmental Science Transactions of the Royal Society of Edinburgh, vol. 46, no. 2, pp. 253–281, 1909. https://doi.org/10.1017/ S0080456800002751
[6] W. Heisenberg, “Über quantentheoretische umdeutung kinematischer und mechanischer beziehungen,” in Original Scientific Papers Wissenschaftliche Originalarbeiten. Springer, 1985, pp. 382–396. https://doi.org/10.1007/BF01328377
[7] H. Weyl, “Gruppentheorie und quantenmechanik,” Zeitschrift für Physik, no. 46, pp. 1–46, 1928. https://doi.org/10.1007/BF02055756
[8] L. Hellstrom and S. Silvestrov, Commuting elements in q-deformed Heisenberg algebras. World Scientific, 2000.
[9] C. Walton, “An invitation to noncommutative algebra,” in A Celebration of the EDGE Program’s Impact on the Mathematics Community and Beyond. Springer, 2019, pp. 339–366. https://arxiv.org/pdf/1808.03172.pdf
[10] A. Reyes and J. Jaramillo, “Symmetry and reversibility properties for quantum algebras and skew poincaré birkhoff-witt extensions,” Ingeniería y Ciencia, vol. 14, no. 27, pp. 29–52, 2018. https://doi.org/10.17230/ ingciencia.14.27.2
[11] S. A. Lopes and F. Razavinia, “Quantum generalized heisenberg algebras and their representations,” arXiv preprint arXiv:2004.09301, 2020. https://arxiv.org/pdf/2004.09301.pdf
[12] M. Tuval and A. Yahalom, “Newton’s third law in the framework of special relativity,” The European Physical Journal Plus, vol. 129, no. 11, pp. 1–8, 2014. https://arxiv.org/pdf/1302.2537v1.pdf
[13] F. B. Kneubil, “Breaking newton’s third law: electromagnetic instances,” European Journal of Physics, vol. 37, no. 6, p. 065201, 2016. https: //doi.org/10.1088/0143-0807/37/6/065201
[14] V. Kac, “Ch. pokman,” Quantum Calculus, Springer–Verlag, New York, 2002.
[15] V. V. Zharinov, “On derivations of the heisenberg algebra,” Theoretical and mathematical physics, vol. 118, no. 2, pp. 129–151, 1999. https: //doi.org/10.1007/BF02557307
[16] B. J. Rana, R. Ahmed, and S. Ahmmed, “Effects of variable electrical conductivity and thermal conductivity on unsteady mhd free convection flow past an exponential accelerated inclined plate,” in AIP Conference Proceedings, vol. 1851, no. 1. AIP Publishing LLC, 2017, p. 020058. https://doi.org/10.1063/1.4984687