Álgebra óptima y soluciones invariantes para la ecuación de Chazy
Main Article Content
Keywords
Ecuación de Chazy, grupo de simetrías de Lie, álgebra óptima, sistema óptimo, soluciones invariantes
Resumen
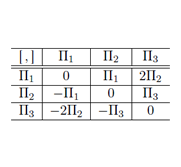
Se caracterizan las soluciones invariantes para la ecuación de Chazy a partir de los operadores generadores del álgebra óptima, la cual fue obtenida mediante el grupo de simetrías de Lie correspondiente a dicha ecuación.
Descargas
Los datos de descargas todavía no están disponibles.
Referencias
[1] S. Lie, “Theorie der transformationsgruppen,” Mathematische Annalen, vol. 2, 1970.
[2] E. Noether, “Invariante variationsprobleme. nachrichten der königlichen gessellschaft der wissenschaften. mathematisch-physikalishe klasse 2, 235–257,” Transport Theory and Statistical Physics, pp. 183–207, 1918.
[3] N. H. Ibragimov, CRC handbook of Lie group analysis of differential equations. CRC press, 1995, vol. 3.
[4] A. Paliathanasis and P. Leach, “Symmetries and singularities of the szekeres system,” Physics Letters A, vol. 381, no. 15, p. 1277–1280, Apr 2017.
[5] A. Ghose-Choudhury, P. Guha, A. Paliathanasis, and P. G. L. Leach, “Noetherian symmetries of noncentral forces with drag term,” International Journal of Geometric Methods in Modern Physics, vol. 14, no. 02, p. 1750018, Jan 2017.
[6] W. Hu, Z. Wang, Y. Zhao, and Z. Deng, “Symmetry breaking of infinitedimensional dynamic system,” Applied Mathematics Letters, vol. 103, p. 106207, 2020.
[7] E. Alimirzaluo, M. Nadjafikhah, and J. Manafian, “Some new exact solutions of $(3+1)$-dimensional burgers system via lie symmetry analysis,” 2021.
[8] A. Paliathanasis, “Lie symmetry analysis and one-dimensional optimal system for the generalized 2+1 kadomtsev-petviashvili equation,” Physica Scripta, vol. 95, no. 5, p. 055223, 2020.
[9] H. Lu and Y. Zhang, “Lie symmetry analysis, exact solutions, conservation laws and bäcklund transformations of the gibbons-tsarev equation,” Symmetry, vol. 12, no. 8, p. 1378, 2020.
[10] S.-F. Tian, “Lie symmetry analysis, conservation laws and solitary wave solutions to a fourth-order nonlinear generalized boussinesq water wave equation,” Applied Mathematics Letters, vol. 100, p. 106056, 2020.
[11] M. R. Ali and R. Sadat, “Lie symmetry analysis, new group invariant for the (3+1)-dimensional and variable coefficients for liquids with gas bubbles models,” Chinese Journal of Physics, 2021.
[12] L. Rosenhead, Laminar Boundary Layers. Clarendon Press, (1963).
[13] P. J. Olver, “Applications of Lie groups to differential equations,” Gradua-te texts in Mathematics, vol. 107 (1993), 1993.
[14] H. Schlichting, “Laminare strahlausbreitung,” Z. Angew. Math. Mech., vol. 13, pp. 260–263, 1933. https://doi.org/10.1002/zamm.19330130403
[15] W. G. Bickley, “The plane jet,” Phil. Mag., vol. 23, pp. 727–721, 1937.
[16] H. B. Squire, 50 fahre Grenzschichtforschung, vol. (1955).
[17] M. B. Glauert, “The wall jet,” J. Fluid Mech., vol. 1, p. 625–643, 1956.
[18] N. Riley, “Asymptotic expansions in radial jets,” J.Math. and Phys., vol. 41, pp. 132–146, 1962. https://doi.org/10.1002/sapm1962411132
[19] L. J. Crane, “Flow past a stretching plate,” Z. Angew. Math. Mech., vol. 21, p. 645–647, 1970. https://doi.org/10.1007/BF01587695
[20] N. H. Ibragimov and M. C. Nucci, “Integration of third order ordinary differential equations by Lie’s method: Equations admitting three-dimensional Lie algebras,” Lie Groups and their applications, vol. 1, pp. 49–64, 1994.
[21] F. M. Mahomed, “Symmetry group classification of ordinary differential equations: Survey of some results,” Mathematical Methods in the Applied Sciences, vol. 30, p. 1995–2012, 2007. https://doi.org/10.1002/mma.934
[22] J. Chazy, “Sur les equations differentielles dont l’integrale generale est uniforme et admet des singelarities essentielles mobiles,” C. R. Acad. Sc. Paris, vol. 149, p. 563–565, 1909.
[23] ——, “Sur les equations differentielles dont l’integrale generale possede une coupure essentielle mobile,” C. R. Acad. Sc. Paris, vol. 150, p. 456–458, 1910.
[24] ——, “Sur les equations differentielles du troisieme ordre et d’ordre superieur dont l’integrale generale a ses points critiques fixes,” Acta Math., vol. 34, p. 317–385, 1911.
[25] P. A. Clarkson and P. J. Olver, “Symmetry and the Chazy equation,” J. Diff. Eq., vol. 124, p. 225–246, 1996. https://www-users.math.umn.edu/ ~olver/s_/chazy.pdf
[2] E. Noether, “Invariante variationsprobleme. nachrichten der königlichen gessellschaft der wissenschaften. mathematisch-physikalishe klasse 2, 235–257,” Transport Theory and Statistical Physics, pp. 183–207, 1918.
[3] N. H. Ibragimov, CRC handbook of Lie group analysis of differential equations. CRC press, 1995, vol. 3.
[4] A. Paliathanasis and P. Leach, “Symmetries and singularities of the szekeres system,” Physics Letters A, vol. 381, no. 15, p. 1277–1280, Apr 2017.
[5] A. Ghose-Choudhury, P. Guha, A. Paliathanasis, and P. G. L. Leach, “Noetherian symmetries of noncentral forces with drag term,” International Journal of Geometric Methods in Modern Physics, vol. 14, no. 02, p. 1750018, Jan 2017.
[6] W. Hu, Z. Wang, Y. Zhao, and Z. Deng, “Symmetry breaking of infinitedimensional dynamic system,” Applied Mathematics Letters, vol. 103, p. 106207, 2020.
[7] E. Alimirzaluo, M. Nadjafikhah, and J. Manafian, “Some new exact solutions of $(3+1)$-dimensional burgers system via lie symmetry analysis,” 2021.
[8] A. Paliathanasis, “Lie symmetry analysis and one-dimensional optimal system for the generalized 2+1 kadomtsev-petviashvili equation,” Physica Scripta, vol. 95, no. 5, p. 055223, 2020.
[9] H. Lu and Y. Zhang, “Lie symmetry analysis, exact solutions, conservation laws and bäcklund transformations of the gibbons-tsarev equation,” Symmetry, vol. 12, no. 8, p. 1378, 2020.
[10] S.-F. Tian, “Lie symmetry analysis, conservation laws and solitary wave solutions to a fourth-order nonlinear generalized boussinesq water wave equation,” Applied Mathematics Letters, vol. 100, p. 106056, 2020.
[11] M. R. Ali and R. Sadat, “Lie symmetry analysis, new group invariant for the (3+1)-dimensional and variable coefficients for liquids with gas bubbles models,” Chinese Journal of Physics, 2021.
[12] L. Rosenhead, Laminar Boundary Layers. Clarendon Press, (1963).
[13] P. J. Olver, “Applications of Lie groups to differential equations,” Gradua-te texts in Mathematics, vol. 107 (1993), 1993.
[14] H. Schlichting, “Laminare strahlausbreitung,” Z. Angew. Math. Mech., vol. 13, pp. 260–263, 1933. https://doi.org/10.1002/zamm.19330130403
[15] W. G. Bickley, “The plane jet,” Phil. Mag., vol. 23, pp. 727–721, 1937.
[16] H. B. Squire, 50 fahre Grenzschichtforschung, vol. (1955).
[17] M. B. Glauert, “The wall jet,” J. Fluid Mech., vol. 1, p. 625–643, 1956.
[18] N. Riley, “Asymptotic expansions in radial jets,” J.Math. and Phys., vol. 41, pp. 132–146, 1962. https://doi.org/10.1002/sapm1962411132
[19] L. J. Crane, “Flow past a stretching plate,” Z. Angew. Math. Mech., vol. 21, p. 645–647, 1970. https://doi.org/10.1007/BF01587695
[20] N. H. Ibragimov and M. C. Nucci, “Integration of third order ordinary differential equations by Lie’s method: Equations admitting three-dimensional Lie algebras,” Lie Groups and their applications, vol. 1, pp. 49–64, 1994.
[21] F. M. Mahomed, “Symmetry group classification of ordinary differential equations: Survey of some results,” Mathematical Methods in the Applied Sciences, vol. 30, p. 1995–2012, 2007. https://doi.org/10.1002/mma.934
[22] J. Chazy, “Sur les equations differentielles dont l’integrale generale est uniforme et admet des singelarities essentielles mobiles,” C. R. Acad. Sc. Paris, vol. 149, p. 563–565, 1909.
[23] ——, “Sur les equations differentielles dont l’integrale generale possede une coupure essentielle mobile,” C. R. Acad. Sc. Paris, vol. 150, p. 456–458, 1910.
[24] ——, “Sur les equations differentielles du troisieme ordre et d’ordre superieur dont l’integrale generale a ses points critiques fixes,” Acta Math., vol. 34, p. 317–385, 1911.
[25] P. A. Clarkson and P. J. Olver, “Symmetry and the Chazy equation,” J. Diff. Eq., vol. 124, p. 225–246, 1996. https://www-users.math.umn.edu/ ~olver/s_/chazy.pdf
Article Sidebar
Publicado
may 12, 2021
Article Details
Cómo citar
Loaiza, G., Acevedo-Agudelo, Y., & Londoño-Duque, O. (2021). Álgebra óptima y soluciones invariantes para la ecuación de Chazy. Ingeniería Y Ciencia, 17(33), 7–21. https://doi.org/10.17230/ingciencia.17.33.1
Número
Sección
Artículos
Agencias de apoyo
Ninguna
Los autores que publican en esta revista están de acuerdo con los siguientes términos:
- Los autores conservan los derechos de autor y garantizan a la revista el derecho de ser la primera publicación del trabajo al igual que licenciado bajo una Creative Commons Attribution License que permite a otros compartir el trabajo con un reconocimiento de la autoría del trabajo y la publicación inicial en esta revista.
- Los autores pueden establecer por separado acuerdos adicionales para la distribución no exclusiva de la versión de la obra publicada en la revista (por ejemplo, situarlo en un repositorio institucional o publicarlo en un libro), con un reconocimiento de su publicación inicial en esta revista.
- Se permite y se anima a los autores a difundir sus trabajos electrónicamente (por ejemplo, en repositorios institucionales o en su propio sitio web) antes y durante el proceso de envío, ya que puede dar lugar a intercambios productivos, así como a una citación más temprana y mayor de los trabajos publicados (Véase The Effect of Open Access) (en inglés).