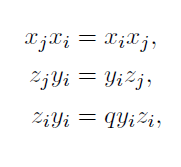A Survey on Some Algebraic Characterizations of Hilbert’s Nullstellensatz for Non-commutative Rings of Polynomial Type
Main Article Content
Keywords
Hilbert’s Nullstellensatz, skew PBW extension, Jacobson ring, generic flatness
Abstract
In this paper we present a survey of some algebraic characterizations of Hilbert’s Nullstellensatz for non-commutative rings of polynomial type. Using several results established in the literature, we obtain a version of this theorem for the skew Poincaré-Birkhoff-Witt extensions. Once this is done, we illustrate the Nullstellensatz with examples appearing in noncommutative ring theory and non-commutative algebraic geometry.
Downloads
References
[1] D. Cox, J. Little, and D. O’Shea, Ideals, Varieties, and Algoritms. An Introduction to Computational Algebraic Geometry and Conmutative Algebra. Springer, 2015.
[2] M. Atiyah and I. G. Macdonald, Introduction to Commutative Algebra. Westview Press, Boulder, CO: Addison-Wesley Series in Mathematics, 1969.
[3] R. S. Irving, “Generic flatness and the Nullstellensatz for Ore extensions,” Comm. Algebra, vol. 7, no. 3, pp. 259–277, 1979. https://doi.org/10.1080/00927877908822347
[4] O. Ore, “Theory of non-commutative polynomials,” Ann. of Maths, vol. 34, no. 3, pp. 480–508, 1933. https://doi.org/10.2307/1968173
[5] K. R. Goodearl and R. B. Warfield, An Introduction to Noncommutative Noetherian Rings, 2nd ed. London: London Mathematical Society Student Texts, 2004.
[6] J. McConnell and J. C. Robson, Noncommutative Noetherian rings. Graduate Studies in Mathematics, AMS, 2001.
[7] R. S. Irving, “Noetherian algebras and the nullstellensatz,” in Séminaire d’Algèbre Paul Dubreil, M.-P. Malliavin, Ed., vol. 740. Berlin, Heidelberg: Springer Berlin Heidelberg, 1979, pp. 80–87. https://doi.org/10.1007/BFb0071054
[8] K. R. Pearson and W. Stephenson, “A skew polynomial ring over a Jacobson ring need not be a Jacobson ring,” Comm. Algebra, vol. 5, no. 8, pp. 783–794, 1977. https://doi.org/10.1080/00927877708822194
[9] A. R. Nasr-Isfahani and A. Moussavi, “Ore extensions of Jacobson rings,” Journal of Algebra, vol. 415, pp. 234 – 246, 2014. https://doi.org/10.1016/j.jalgebra.2014.06.011
[10] M. Duflo, “Certaines algebres de type fini sont des algebres de Jacobson,” J. Algebra, vol. 27, no. 2, pp. 358–365, 1973. https://doi.org/10.1016/0021-8693(73)90110-5
[11] J. McConnell and J. Robson, “The Nullstellensatz and generic flatness,” Perspectives in Ring Theory, pp. 227–232, 1988. https://doi.org/10.1007/978-94-009-2985-2_19
[12] M. Artin, L. W. Small, and J. J. Zhang, “Generic flatness for strongly Noetherian algebras,” J. Algebra, vol. 221, no. 2, pp. 579–610, 1999. https://doi.org/10.1006/jabr.1999.7997
[13] H. Suárez, O. Lezama, and A. Reyes, “Calabi–Yau property for graded skew PBW extensions,” Rev. Colombiana Mat., vol. 51, no. 2, pp. 221–238, 2017. http://www.scielo.org.co/scielo.php?script=sci_arttext&pid=S0034-74262017000200221&nrm=iso
[14] E. Hashemi, K. Khalilnezhad, and A. Alhevaz, “(_; _)-compatible skew PBW extension ring,” Kyungpook Math. J., vol. 57, no. 3, pp. 401–417, 2017. https://doi.org/10.1007/s00006-017-0800-4
[15] E. Hashemi, K. Khalilnezhad, and A. Alhevaz, “Extensions of rings over 2-primal rings,” Matematiche (Catania), vol. 74, no. 1, pp. 141–162, 2019. https://doi.org/10.4418/2019.74.1.10
[16] E. Hashemi, K. Khalilnezhad, and H. Ghadiri, “Baer and quasi-Baer properties of skew PBW extensions,” J. Algebr. Syst., vol. 7, no. 1, pp. 1–24, 2019. http://www.scielo.org.co/pdf/rein/v33n2/v33n2a07.pdf
[17] O. Lezama and E. Latorre, “Non-commutative algebraic geometry of semi-graded rings,” Int. J. Algebra Comput., vol. 27, no. 4, pp. 361–389, 2017. https://doi.org/10.1142/S0218196717500199
[18] O. Lezama and H. Venegas, “Some homological properties of skew PBW extensions arising in non-commutative algebraic geometry,” Discuss. Math. Gen. Algebra Appl., vol. 37, no. 1, pp. 45–57, 2017. https://doi.org/10.7151/dmgaa.1264
[19] A. Reyes and H. Suárez, “_-PBW extensions of skew Armendariz rings,” Adv. Appl. Clifford Algebr., vol. 27, no. 4, pp. 3197–3224, 2017. https://doi.org/10.1007/s00006-017-0800-4
[20] A. Reyes and J. Jaramillo, “Symmetry and reversibility properties for quantum algebras and skew Poincaré–Birkhoff–Witt extensions,” Ingeniería y Ciencia, vol. 14, no. 27, pp. 29–52, 2018. https://doi.org/10.17230/ingciencia.14.27.2
[21] H. Suárez and A. Reyes, “Nakayama automorphism of some skew PBW extensions,” Ingeniería y Ciencia, vol. 15, no. 29, pp. 157–177, 2019. https://doi.org/10.17230/ingciencia.15.29.6
[22] A. Reyes and C. Rodríguez, “The McCoy condition on skew PBW extensions,” Commun. Math. Stat., 2019. https://doi.org/10.1007/s40304-019-00184-5
[23] C. Gallego and O. Lezama, “Gröbner bases for ideals of _-PBW extensions,” Comm. Algebra, vol. 39, no. 1, pp. 50–75, 2011. https://doi.org/10.1080/00927870903431209
[24] A. Bell and K. Goodearl, “Uniform rank over differential operator rings and Poincaré–Birkhoff–Witt extensions,” Pacific J. Math., vol. 131, no. 1, pp. 13–37, 1988. https://projecteuclid.org/download/pdf_1/euclid.pjm/1102690067
[25] V. A. Artamonov, “Derivations of skew PBW extensions,” Commun.Math. Stat., vol. 3, no. 4, pp. 449–457, 2015. https://doi.org/10.1007/s40304-015-0067-9
[26] C. Gallego and O. Lezama, “d-hermite rings and skew PBW extensions,” São Paulo J. Math. Sci., vol. 10, no. 1, pp. 60–72, 2016. https://doi.org/10.1007/s40863-015-0010-8
[27] A. Niño and A. Reyes, “Some ring theoretical properties of skew Poincaré–Birkhoff–Witt extensions,” Bol. Mat., vol. 24, no. 2, pp. 131–148, 2017. https://dialnet.unirioja.es/servlet/articulo?codigo=6332586
[28] A. Reyes, “Armendariz modules over skew PBW extensions,” Comm. Algebra, vol. 47, no. 3, pp. 1248–1270, 2019. https://doi.org/10.1080/00927872.2018.1503281
[29] A. Reyes and H. Suárez, “A note on zip and reversible skew PBW extensions,” Bol. Mat., vol. 23, no. 1, pp. 71–79, 2017. https://pdfs.semanticscholar.org/a707/8bf0bc39d52dcbfcb22b383a460afcd6eeb8.pdf
[30] A. Reyes and H. Suárez, “Skew Poincaré–Birkhoff–Witt extensions over weak zip rings,” Beitr. Algebra Geom., vol. 60, no. 2, pp. 197–216, 2019. https://doi.org/10.1007/s13366-018-0412-8
[31] O. Lezama and A. Reyes, “Some homological properties of skew PBW extensions,” Comm. Algebra, vol. 42, pp. 1200–1230, 2014. https://doi.org/10.1080/00927872.2012.735304
[32] A. Reyes and H. Suárez, “Radicals and Köthe’s conjecture for skew PBW extensions,” Commun. Math. Stat, 2019. https://doi.org/10.1007/s40304-019-00189-0
[33] A. Reyes and Y. Suárez, “On the ACCP in skew Poincaré–Birkhoff–Witt extensions,” Beitr. Algebra Geom., vol. 59, no. 4, pp. 625–643, 2018. https://doi.org/10.1007/s13366-018-0384-8
[34] A. Reyes and H. Suárez, “PBW bases for some 3-dimensional skew polynomial algebras,” Far East J. Math. Sci. (FJMS), vol. 101, no. 6, pp. 1207–1228, 2017. https://arxiv.org/pdf/1805.03489v1.pdf
[35] A. Reyes and H. Suárez, “Some remarks about the cyclic homology of skew PBW extensions,” Ciencia en Desarrollo, vol. 7, no. 2, pp. 99–107, 2016. http://www.scielo.org.co/scielo.php?script=sci_arttext&pid=S0121-74882016000200009&nrm=iso
[36] A. Reyes and H. Suárez, “Bases for quantum algebras and skew Poincaré–Birkhoff–Witt extensions,”
Momento, vol. 54, no. 2, pp. 54–75, 2017. http://www.scielo.org.co/scielo.php?script=sci_arttext&pid=S0121-44702017000100005&nrm=iso 42
[37] J. Dixmier, Enveloping Algebras, 2nd ed. Graduate Studies in Mathematics, 1977.
[38] H. Li, Noncommutative Gröbner Bases and Filtered-Graded Transfer. Springer, 2002.
[39] A. Rosenberg, Noncommutative Algebraic Geometry and Representations of Quantized Algebras, 2nd ed. Mathematics and its Applications, 330 Kluwer Academic Publishers, 1995.
[40] S. Woronowicz, “Twisted SU(2) group. An example of a non-commutative differential calculus,” Publ. Res. Inst. Math. Sci., vol. 23, no. 1, pp. 117–181, 1987. https://doi.org/10.2977/prims/1195176848
[41] W. Jategaonkar, “A multiplicative analog of the Weyl algebra,” Comm. Algebra, vol. 12, no. 14, pp. 1669–1688, 1984. https://doi.org/10.1080/00927878408823074
[42] M. Havlícek, A. A. U. Klimyk, and S. Pošta, “Central elements of the algebras U0q (som) and Uq(isom),” Czechoslovak Journal of Physics, vol. 50, no. 1, p. Springer, 2000. https://doi.org/10.1023/A:1022825031633
[43] N. Iorgov, “On the center of q-deformed algebra U0q (so3) related to quantum gravity at q root of 1,” Conf, vol. 43, no. 2, pp. 449–455, 2002. https://www.slac.stanford.edu/econf/C0107094/papers/Iorgov449-455.pdf
[44] R. Berger, “The Quantum Poincaré-Birkhoff-Witt theorem,” Comm. Math. Phys., vol. 143, no. 2, pp. 215–234, 1992. https://doi.org/10.1007/BF02099007
[45] D. Jordan, “The graded algebra generated by two eulerian derivatives,” Algebr. Represent. Theory, vol. 4, no. 3, pp. 249–275, 2001.