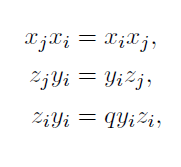Un estudio sobre algunas caracterizaciones algebraicas del teorema de ceros de Hilbert para anillos no conmutativos de tipo polinomial
Main Article Content
Keywords
Teorema de ceros de Hilbert, extensión PBW torcida, anillo de Jacobson, plenitud genérica
Resumen
En este artículo presentamos un estudio sobre algunas caracterizaciones algebraicas del teorema de Nullstellensatz de Hilbert para anillos no conmutativos de tipo polinomial. Utilizando varios resultados establecidos en la literatura, obtuvimos una versión de este teorema para las extensiones de Poincaré-Birkhoff-Witt. Una vez hecho esto, ilustramos el Nullstellensatz con ejemplos que aparecen en la teoría de los anillos no conmutativa y en la geometría algebraica no conmutativa.
Descargas
Referencias
[1] D. Cox, J. Little, and D. O’Shea, Ideals, Varieties, and Algoritms. An Introduction to Computational Algebraic Geometry and Conmutative Algebra. Springer, 2015.
[2] M. Atiyah and I. G. Macdonald, Introduction to Commutative Algebra. Westview Press, Boulder, CO: Addison-Wesley Series in Mathematics, 1969.
[3] R. S. Irving, “Generic flatness and the Nullstellensatz for Ore extensions,” Comm. Algebra, vol. 7, no. 3, pp. 259–277, 1979. https://doi.org/10.1080/00927877908822347
[4] O. Ore, “Theory of non-commutative polynomials,” Ann. of Maths, vol. 34, no. 3, pp. 480–508, 1933. https://doi.org/10.2307/1968173
[5] K. R. Goodearl and R. B. Warfield, An Introduction to Noncommutative Noetherian Rings, 2nd ed. London: London Mathematical Society Student Texts, 2004.
[6] J. McConnell and J. C. Robson, Noncommutative Noetherian rings. Graduate Studies in Mathematics, AMS, 2001.
[7] R. S. Irving, “Noetherian algebras and the nullstellensatz,” in Séminaire d’Algèbre Paul Dubreil, M.-P. Malliavin, Ed., vol. 740. Berlin, Heidelberg: Springer Berlin Heidelberg, 1979, pp. 80–87. https://doi.org/10.1007/BFb0071054
[8] K. R. Pearson and W. Stephenson, “A skew polynomial ring over a Jacobson ring need not be a Jacobson ring,” Comm. Algebra, vol. 5, no. 8, pp. 783–794, 1977. https://doi.org/10.1080/00927877708822194
[9] A. R. Nasr-Isfahani and A. Moussavi, “Ore extensions of Jacobson rings,” Journal of Algebra, vol. 415, pp. 234 – 246, 2014. https://doi.org/10.1016/j.jalgebra.2014.06.011
[10] M. Duflo, “Certaines algebres de type fini sont des algebres de Jacobson,” J. Algebra, vol. 27, no. 2, pp. 358–365, 1973. https://doi.org/10.1016/0021-8693(73)90110-5
[11] J. McConnell and J. Robson, “The Nullstellensatz and generic flatness,” Perspectives in Ring Theory, pp. 227–232, 1988. https://doi.org/10.1007/978-94-009-2985-2_19
[12] M. Artin, L. W. Small, and J. J. Zhang, “Generic flatness for strongly Noetherian algebras,” J. Algebra, vol. 221, no. 2, pp. 579–610, 1999. https://doi.org/10.1006/jabr.1999.7997
[13] H. Suárez, O. Lezama, and A. Reyes, “Calabi–Yau property for graded skew PBW extensions,” Rev. Colombiana Mat., vol. 51, no. 2, pp. 221–238, 2017. http://www.scielo.org.co/scielo.php?script=sci_arttext&pid=S0034-74262017000200221&nrm=iso
[14] E. Hashemi, K. Khalilnezhad, and A. Alhevaz, “(_; _)-compatible skew PBW extension ring,” Kyungpook Math. J., vol. 57, no. 3, pp. 401–417, 2017. https://doi.org/10.1007/s00006-017-0800-4
[15] E. Hashemi, K. Khalilnezhad, and A. Alhevaz, “Extensions of rings over 2-primal rings,” Matematiche (Catania), vol. 74, no. 1, pp. 141–162, 2019. https://doi.org/10.4418/2019.74.1.10
[16] E. Hashemi, K. Khalilnezhad, and H. Ghadiri, “Baer and quasi-Baer properties of skew PBW extensions,” J. Algebr. Syst., vol. 7, no. 1, pp. 1–24, 2019. http://www.scielo.org.co/pdf/rein/v33n2/v33n2a07.pdf
[17] O. Lezama and E. Latorre, “Non-commutative algebraic geometry of semi-graded rings,” Int. J. Algebra Comput., vol. 27, no. 4, pp. 361–389, 2017. https://doi.org/10.1142/S0218196717500199
[18] O. Lezama and H. Venegas, “Some homological properties of skew PBW extensions arising in non-commutative algebraic geometry,” Discuss. Math. Gen. Algebra Appl., vol. 37, no. 1, pp. 45–57, 2017. https://doi.org/10.7151/dmgaa.1264
[19] A. Reyes and H. Suárez, “_-PBW extensions of skew Armendariz rings,” Adv. Appl. Clifford Algebr., vol. 27, no. 4, pp. 3197–3224, 2017. https://doi.org/10.1007/s00006-017-0800-4
[20] A. Reyes and J. Jaramillo, “Symmetry and reversibility properties for quantum algebras and skew Poincaré–Birkhoff–Witt extensions,” Ingeniería y Ciencia, vol. 14, no. 27, pp. 29–52, 2018. https://doi.org/10.17230/ingciencia.14.27.2
[21] H. Suárez and A. Reyes, “Nakayama automorphism of some skew PBW extensions,” Ingeniería y Ciencia, vol. 15, no. 29, pp. 157–177, 2019. https://doi.org/10.17230/ingciencia.15.29.6
[22] A. Reyes and C. Rodríguez, “The McCoy condition on skew PBW extensions,” Commun. Math. Stat., 2019. https://doi.org/10.1007/s40304-019-00184-5
[23] C. Gallego and O. Lezama, “Gröbner bases for ideals of _-PBW extensions,” Comm. Algebra, vol. 39, no. 1, pp. 50–75, 2011. https://doi.org/10.1080/00927870903431209
[24] A. Bell and K. Goodearl, “Uniform rank over differential operator rings and Poincaré–Birkhoff–Witt extensions,” Pacific J. Math., vol. 131, no. 1, pp. 13–37, 1988. https://projecteuclid.org/download/pdf_1/euclid.pjm/1102690067
[25] V. A. Artamonov, “Derivations of skew PBW extensions,” Commun.Math. Stat., vol. 3, no. 4, pp. 449–457, 2015. https://doi.org/10.1007/s40304-015-0067-9
[26] C. Gallego and O. Lezama, “d-hermite rings and skew PBW extensions,” São Paulo J. Math. Sci., vol. 10, no. 1, pp. 60–72, 2016. https://doi.org/10.1007/s40863-015-0010-8
[27] A. Niño and A. Reyes, “Some ring theoretical properties of skew Poincaré–Birkhoff–Witt extensions,” Bol. Mat., vol. 24, no. 2, pp. 131–148, 2017. https://dialnet.unirioja.es/servlet/articulo?codigo=6332586
[28] A. Reyes, “Armendariz modules over skew PBW extensions,” Comm. Algebra, vol. 47, no. 3, pp. 1248–1270, 2019. https://doi.org/10.1080/00927872.2018.1503281
[29] A. Reyes and H. Suárez, “A note on zip and reversible skew PBW extensions,” Bol. Mat., vol. 23, no. 1, pp. 71–79, 2017. https://pdfs.semanticscholar.org/a707/8bf0bc39d52dcbfcb22b383a460afcd6eeb8.pdf
[30] A. Reyes and H. Suárez, “Skew Poincaré–Birkhoff–Witt extensions over weak zip rings,” Beitr. Algebra Geom., vol. 60, no. 2, pp. 197–216, 2019. https://doi.org/10.1007/s13366-018-0412-8
[31] O. Lezama and A. Reyes, “Some homological properties of skew PBW extensions,” Comm. Algebra, vol. 42, pp. 1200–1230, 2014. https://doi.org/10.1080/00927872.2012.735304
[32] A. Reyes and H. Suárez, “Radicals and Köthe’s conjecture for skew PBW extensions,” Commun. Math. Stat, 2019. https://doi.org/10.1007/s40304-019-00189-0
[33] A. Reyes and Y. Suárez, “On the ACCP in skew Poincaré–Birkhoff–Witt extensions,” Beitr. Algebra Geom., vol. 59, no. 4, pp. 625–643, 2018. https://doi.org/10.1007/s13366-018-0384-8
[34] A. Reyes and H. Suárez, “PBW bases for some 3-dimensional skew polynomial algebras,” Far East J. Math. Sci. (FJMS), vol. 101, no. 6, pp. 1207–1228, 2017. https://arxiv.org/pdf/1805.03489v1.pdf
[35] A. Reyes and H. Suárez, “Some remarks about the cyclic homology of skew PBW extensions,” Ciencia en Desarrollo, vol. 7, no. 2, pp. 99–107, 2016. http://www.scielo.org.co/scielo.php?script=sci_arttext&pid=S0121-74882016000200009&nrm=iso
[36] A. Reyes and H. Suárez, “Bases for quantum algebras and skew Poincaré–Birkhoff–Witt extensions,”
Momento, vol. 54, no. 2, pp. 54–75, 2017. http://www.scielo.org.co/scielo.php?script=sci_arttext&pid=S0121-44702017000100005&nrm=iso 42
[37] J. Dixmier, Enveloping Algebras, 2nd ed. Graduate Studies in Mathematics, 1977.
[38] H. Li, Noncommutative Gröbner Bases and Filtered-Graded Transfer. Springer, 2002.
[39] A. Rosenberg, Noncommutative Algebraic Geometry and Representations of Quantized Algebras, 2nd ed. Mathematics and its Applications, 330 Kluwer Academic Publishers, 1995.
[40] S. Woronowicz, “Twisted SU(2) group. An example of a non-commutative differential calculus,” Publ. Res. Inst. Math. Sci., vol. 23, no. 1, pp. 117–181, 1987. https://doi.org/10.2977/prims/1195176848
[41] W. Jategaonkar, “A multiplicative analog of the Weyl algebra,” Comm. Algebra, vol. 12, no. 14, pp. 1669–1688, 1984. https://doi.org/10.1080/00927878408823074
[42] M. Havlícek, A. A. U. Klimyk, and S. Pošta, “Central elements of the algebras U0q (som) and Uq(isom),” Czechoslovak Journal of Physics, vol. 50, no. 1, p. Springer, 2000. https://doi.org/10.1023/A:1022825031633
[43] N. Iorgov, “On the center of q-deformed algebra U0q (so3) related to quantum gravity at q root of 1,” Conf, vol. 43, no. 2, pp. 449–455, 2002. https://www.slac.stanford.edu/econf/C0107094/papers/Iorgov449-455.pdf
[44] R. Berger, “The Quantum Poincaré-Birkhoff-Witt theorem,” Comm. Math. Phys., vol. 143, no. 2, pp. 215–234, 1992. https://doi.org/10.1007/BF02099007
[45] D. Jordan, “The graded algebra generated by two eulerian derivatives,” Algebr. Represent. Theory, vol. 4, no. 3, pp. 249–275, 2001.
Article Sidebar
Article Details
Armando Reyes, Universidad Nacional de Colombia
Doctor en Ciencias Matemáticas. Profesor del Departamento de Matemáticas, Universidad Nacional de Colombia, sede Bogotá. Ver perfil en Google Académico
Jason Hernández-Mogollón, Universidad Nacional de Colombia
Magíster en Matemáticas. Estudiante de Doctorado en Ciencias- Matemáticas en Universidad Nacional de Colombia, sede Bogotá.
Los autores que publican en esta revista están de acuerdo con los siguientes términos:
- Los autores conservan los derechos de autor y garantizan a la revista el derecho de ser la primera publicación del trabajo al igual que licenciado bajo una Creative Commons Attribution License que permite a otros compartir el trabajo con un reconocimiento de la autoría del trabajo y la publicación inicial en esta revista.
- Los autores pueden establecer por separado acuerdos adicionales para la distribución no exclusiva de la versión de la obra publicada en la revista (por ejemplo, situarlo en un repositorio institucional o publicarlo en un libro), con un reconocimiento de su publicación inicial en esta revista.
- Se permite y se anima a los autores a difundir sus trabajos electrónicamente (por ejemplo, en repositorios institucionales o en su propio sitio web) antes y durante el proceso de envío, ya que puede dar lugar a intercambios productivos, así como a una citación más temprana y mayor de los trabajos publicados (Véase The Effect of Open Access) (en inglés).